UCAT Quantitative Reasoning Mathematics Topic Book

Understanding The Design

The Quantitative Reasoning subtest allows prospective medical students to demonstrate their ability to solve various numeracy-based problems. Demonstrating good numerical reasoning is incredibly important within medicine where doctors often calculate prescription drug calculations, where medical professionals must factor in their patient’s: weight, height and age in order to maximise drug efficacy whilst staying within the bounds of the therapeutic drug index. In clinical research, being able to interpret, critique and apply statistical data is becoming increasingly important in the practice of Evidence-Based Medicine and holistic healthcare. 

In the Quantitative Reasoning subtest, you will be presented with 36 questions associated with tables, charts and/or graphs which must be completed in 25 minutes. These questions will refer to a short passage of text, charts, tables, graphs or diagrams. Questions in Quantitative Reasoning may be presented as a standalone question, or a series of questions relating to one table, chart and/or graph. All questions will have five multiple choice answer options - where you must decide which is the most accurate response.

How Is Quantitative Reasoning Marked?

In Quantitative Reasoning, you are marked based on the number of correct answers you give, where there are no partial marks available for Quantitative Reasoning. 

Question Types

Simple Arithmetic
The simple arithmetic question type, or rather numeracy-based questions, assess your ability to apply simple numerical functions, such as: addition, subtraction, multiplication and division, onto a related data set. 

Algebra
Algebraic-style questions assess your ability to manipulate equations in order to solve for unknown values, such as basic substitution and equation rearrangement. This question type may require you to generate an equation based off of written information in a passage. 

Geometry
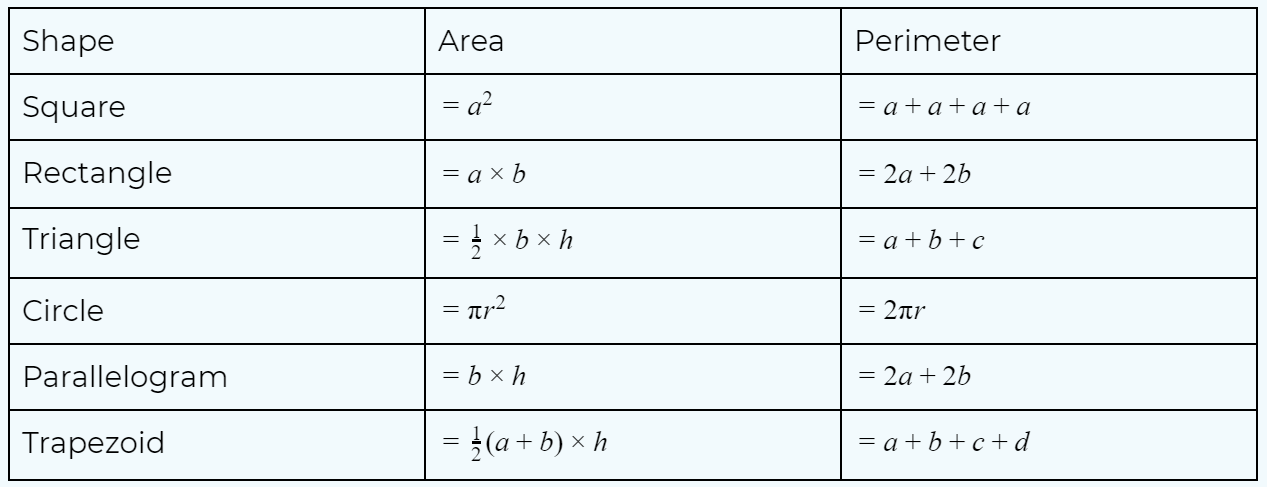
Geometry-style questions require you to be familiar with a handful of prerequisite formulas, such as finding the area or perimeter of a given shape. Geometry-related questions will require you to estimate answers, provide proportions or ratios, or accurately determine the answer across five multiple choice answer options.

Statistics
Statistics in Quantitative Reasoning include mean, mode and median as well as extending to the application of fundamental probability laws which can be presented in the form of text, charts, tables or diagrams. 

Strategic Approach

It is important to note that, in Quantitative Reasoning, numeracy-based questions do not require advanced knowledge. The UCAT Consortium recommends that students lightly revise their mathematical reasoning skills in geometry, area of percentages, proportions and ratios, rates and averages with regard to Quantitative Reasoning.

Percentages

Percentages, fractions and decimals are different ways of conveying information. A percentage, also referred to as per cent (%), is a fraction of a number out of 100. For example 15% equates to ¹⁵⁄₁₀₀.
Example: Percentages

Question 1. 
Calculate the percentage of ²⁰⁄₁₀₀. 

Recognise that a percentage refers to a number out of 100 (the denominator). This fraction is already in its correct form, and thus can be easily converted into its percentage:

Percentage (%) =20

Question 2. 

Calculate the percentage of ²⁵⁄₅₀. 

Recognise that this percentage refers to a number out of 50 (the denominator). We must make the denominator into 100 by multiplying it by 2. Remember, what you do to one side (the denominator), you must do to the other (the numerator). 

Percentage (%) =²⁵⁄₅₀
Percentage (%) =²⁵⁄₅₀  x 50
Percentage (%) =⁵⁰⁄₁₀₀
Percentage (%) =50
Thus, ²⁵⁄₅₀equates to 50%. 

Question 3.
Convert ⁶⁄₈ to a percentage.
Transform the denominator into 100. To do this, we need to know what number, multiplied by 8, gets us to 100. You can solve for this by dividing 100 by 8.

=¹⁰⁰⁄₈
= 12.5

 Now, we must multiply the numerator and the denominator of the fraction by 12.5. 

=⁶⁄₈ x 12.5
=⁷⁵⁄₁₀₀

Recognise that a percentage refers to a number out of 100 (the denominator). This fraction is already in its correct form, and thus can be easily converted into its percentage:

 Percentage (%) =75

Question 4.

Convert 9% to a decimal.
Write the percent as a fraction.
9% =⁹⁄₁₀₀
9% =0.09

Hint: as 9% represents 9 hundreths, to calculate 9% you can simply divide 9 by 100 (or move the decimal point to the left by two decimal places), this will give you 0.09.

Question 5.

Convert 0.55 to a percent. 
Write the percent as a fraction.

0.55 =⁵⁵⁄₁₀₀
0.55 =55%

Hint: To convert 0.55 into a fraction or percentage, you can simply multiply 0.55 by 100 (or move the decimal point to the right by two decimal places), this will give you 55%. 

Quantitative Reasoning may also require you to calculate percentage change. Percentage change represents the degree of change over time. To calculate the percentage change, you must first work out the difference between the two comparator values. Note that the calculations will be different based on whether the value is increasing or decreasing.

Increase=New Value - Original Value 
or
Decrease=Original Value - New Value

Once you have determined the difference, the divide the value by the original number, and then multiply that number by 100.

% increase =^increase⁄_{original value x 100}
or
% decrease =^decrease⁄_{original value x 100}

Proportions and Ratios

A proportion describes an equality between two ratios. Proportions are used to establish ratios and to solve for unknown quantities using arithmetic operations. A ratio, however, compares to values and can be written as a:b or ab. There are two different types of ratios within Quantitative Reasoning, where ratios can be written as part-to-part or part-to-whole, and can be written as:

part : whole = part : sum of all parts

Proportions can only be discerned if a ratio is known and can be written as: a:b=c:d or ^a⁄_b=^c⁄_d. 

Example: Proportions and Ratios

Daniel wants to bake a cake for fathers day; however the recipe creates far too much cake batter. 

Question 1.

The cake recipe uses¹⁄₄ corn flour and14 all-purpose flour. What is the ratio of corn flour to all-purpose flour used in the recipe?

The recipe uses the same amount of all-purpose flour and rice flour, which means the ratio of all-purpose flour to rice flour is 1:1. 

Question 2.

Daniel has six eggs, 2 cups of corn flour, 2 cups of all-purpose flour and 3 cups of milk. What is the ratio of the number of eggs to the remaining ingredients Daniel has?

Note that we are calculating a part-to-whole ratio here. Daniel has a total of 13 ingredients, where eggs constitute 6 of these ingredients. Thus, the ratio is 6:13. 

Rates

A rate is a ratio that uses different units, such as speed (a ratio of distance and time). Rates are normally expressed as fractions, for example: speed=^distance⁄_time. Pearson Vue recommends that UCAT students become well-versed in the speed, distance and time ratio as it is commonly used in the UCAT. 

Example: Rates

Question 1

Question 2

Useful Geometry Formulas

Single Variable Data Analysis

Single variable data analysis refers to descriptive statistics used in data sets, such as: mean, mode, median and range. Mean and median are often referred to as measures of central tendency, where they both measure the centre of the data set. The mean is simply the average of all observations within a set of data and the median is the middle observation within an ordered set of data. 

Mode and range are also useful descriptive statistics within data sets. The mode statistic refers to the most frequently occurring value within a data set. The range, another descriptive statistic, is the difference between the highest and lowest values within a set of data. The range statistic is useful wherein it provides a measure of spread within the data. 

Calculating the Mean, Mode and Median

To calculate the mean (x) of a set of data, all values must be added together divided by the number of values within the data set.

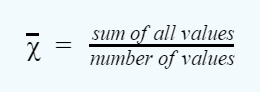
Example: Calculating the Mean, Mode and Median
Daisy, Natasha, Dominic, Andrew and Olivia recently received their scores back from a maths test. Daisy scored a 90, Natasha and Sarah scored a 40, Dominic scored a 99, Andrew scored a 75 and Olivia scored a 67. 

Question 1. 

Calculate the mean test score.
Firstly combine all the test score values.

Sum of All Scores = 90+40+40+99+75+67
Sum of All Scores = 371

Next, count how many values were included. As we included the test scores of Daisy, Natasha, Sarah, Dominic, Andrew and Olivia, we have a total of six values. 

Now, use the equation above to find the mean of this data set.

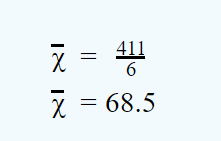
Thus, the mean score on the test was 68.5.

Question 2.

What is the mode statistic of the data set? 

Count how many times a number occurs within the data set. The number that occurs the most times is considered the mode statistic. 

90, 99, 75 and 67 occur only once in the data set. 40 occurs twice (Natasha and Sarah’s test scores). Thus, the mode statistic in this data set is 40.

Question 3. 

What is the median statistic in this data set?

Firstly order all of the test results, from lowest to highest.

40, 40, 67, 75, 90, 99.

Look at the most middle number. In this instance, there are two middle numbers: 67 and 75. To calculate the median, take the average of these two numbers. 

Sum of All Scores = 67+75
Sum of All Scores = 142

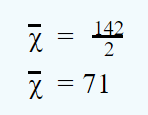
Question 4. 

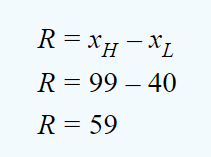
What is the range statistic in this data set?
Take the highest value and subtract it from the lowest value.

How To Improve

Quantitative Reasoning is amongst the easier UCAT subtests, where Quantitative Reasoning has the highest mean score. Fortunately, leverage can be gained through familiarity with equations and mathematical laws in Quantitative Reasoning.

Firstly, it’s important to understand where you are currently placed in Quantitative Reasoning. At Frasers, we recommend that students sit a diagnostic exam prior to any study in order to gain a broad understanding of their score range. From this, we can then understand whether you are sitting in the lower (0 - 40%), middle (40 - 60%) or upper (60 - 100%) percentiles. 

After sitting a diagnostic exam, it is recommended that students review strategies for question types they found challenging, as well as continuing to reinforce and strengthen question types that were easier in contrast. The UCAT Consortium also provides a few strategies available for different question types to help guide your improvement. 

Students who have previously scored well in Quantitative Reasoning recommend avoiding the on-screen calculator, and emphasise being able to mentally calculate the answer especially when the possible answer options are spread widely apart. 

Where To From Here?

The whole intention behind this topic book is to enhance your understanding and preparation experience prior to undertaking the UCAT exam. Besides, this topic book explores the various Quantitative Reasoning question-types, and provides a holistic approach to support your UCAT prep and to ultimately excel in this section.

Alternatively, here’s a list of other useful resources that are free and easily accessible to all students who wish to pursue medicine.

1. What UCAT score is needed for medicine?
2. What is Problem-Based Learning in Medicine?
3. 5 Biggest UCAT Myths Debunked